Making It Clear: The Importance of Transparency
Sandra Lach Arlinghaus
Escher/Barr Earth
| INTRODUCTION Escher's art in the "Circle Limit" series shows interdigitated pattern realized inside a circle with the repeating pattern shrinking as one moves outward from the center of the circle. In his correspondence with the geometer H. S. M. Coxeter, one sees the emergence of these figures. Escher employed the Poincaré Disk representation of hyperbolic geometry to represent repeating pattern veering off to infinity: a compact and bounded infinity rather than the usual unbounded one that lurks in our Euclidean-only trained minds. In "The Animated Pascal," an early Escher screen capture was superimposed on Google Earth, suggesting how a Circle Limit image might look when wrapped around a sphere--much like reprojecting a map back to the globe. While the result is interesing as a visual representation, it does not really show what would happen had the Circle Limit series actually been drawn on a spinning globe. One might suppose, that had such drawing taken place, that all polygons would have had identical size. As one approched what had been the horizon, the seemingly small distant polygons would emerge as full-sized. A change in vantage point often reveals differences in space and time. |
| UNMASKING GOOGLE
EARTH: REVEALING THE GOOGLE GLOBE Consider using the globe underlying Google Earth as a platform to represent a variety of spherical models. Figure 1 illustrates how to remove the Earth-skin, revealing Google Globe. The initial frame of the animation shows Google Earth with continents, oceans, and so forth. The second frame shows the graticule placed on the globe of continents. To activate the graticule, go to View | Grid in the pull-down menus at the top. Finally, remove the continental skin. The third frame shows highlighting of the layer "Primary database" in the Layers box on the left. In the fourth and final frame, the slider in the "Places" box, at the bottom is moved all the way to the left. This action causes the continental skin covering the globe to be obscured. Now, the Google Earth skin, its landmasses and oceans, has been converted to a blank sphere with a graticule (spherical grid). The Grid may be left on or turned off for a totally blank sphere. (For user-control of the animation sequence, click on this link to a movie of the animation sequence in Figure 1.) |
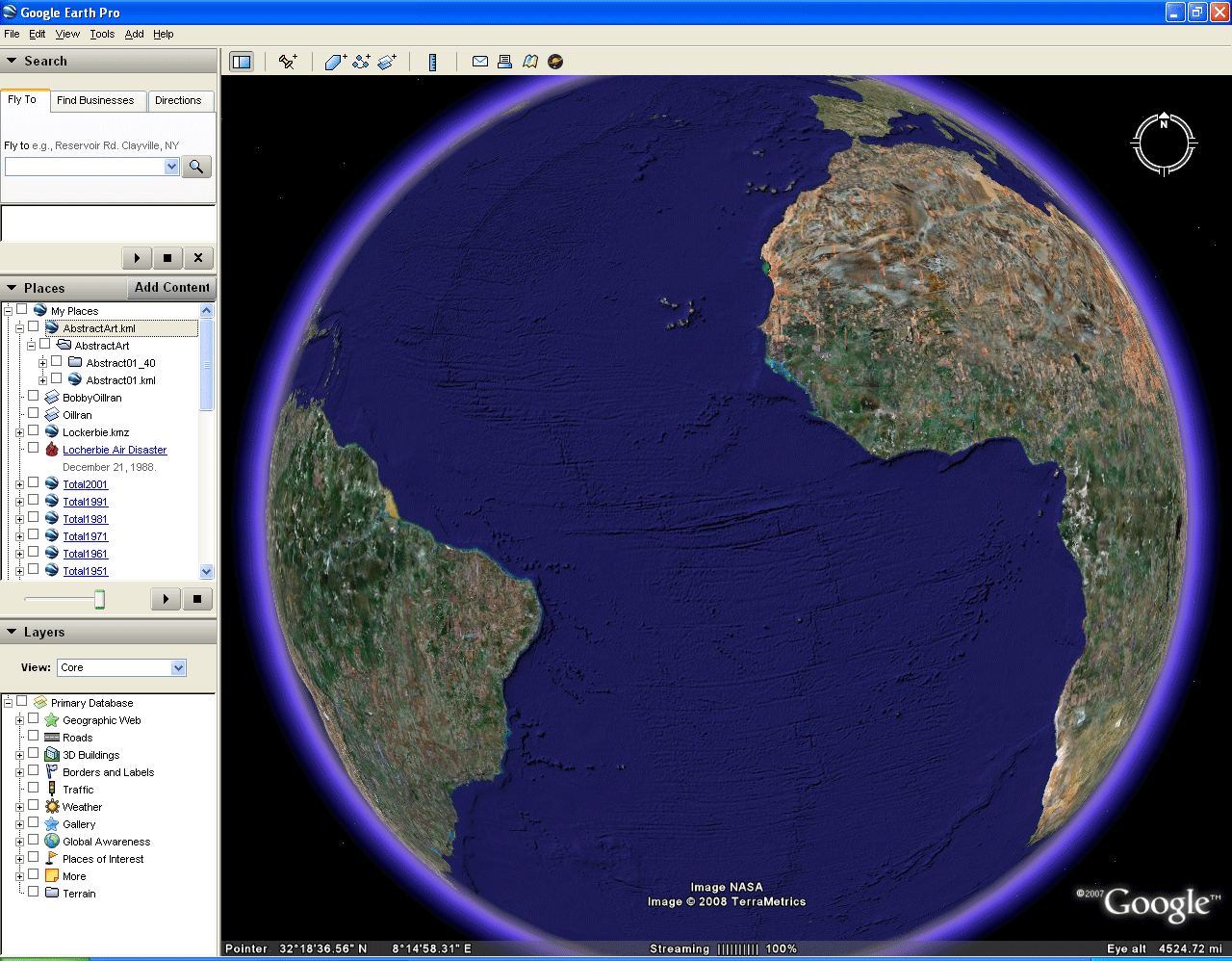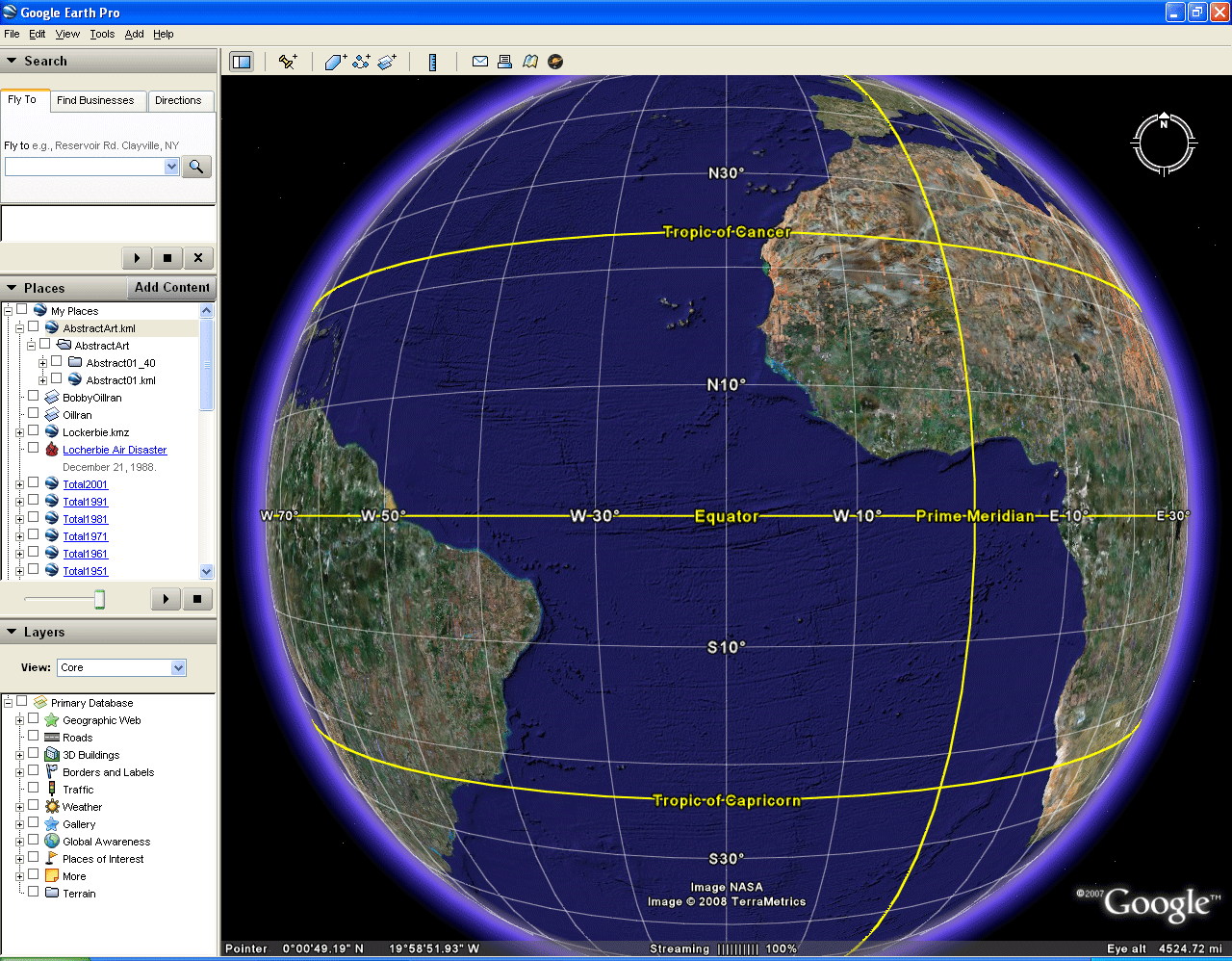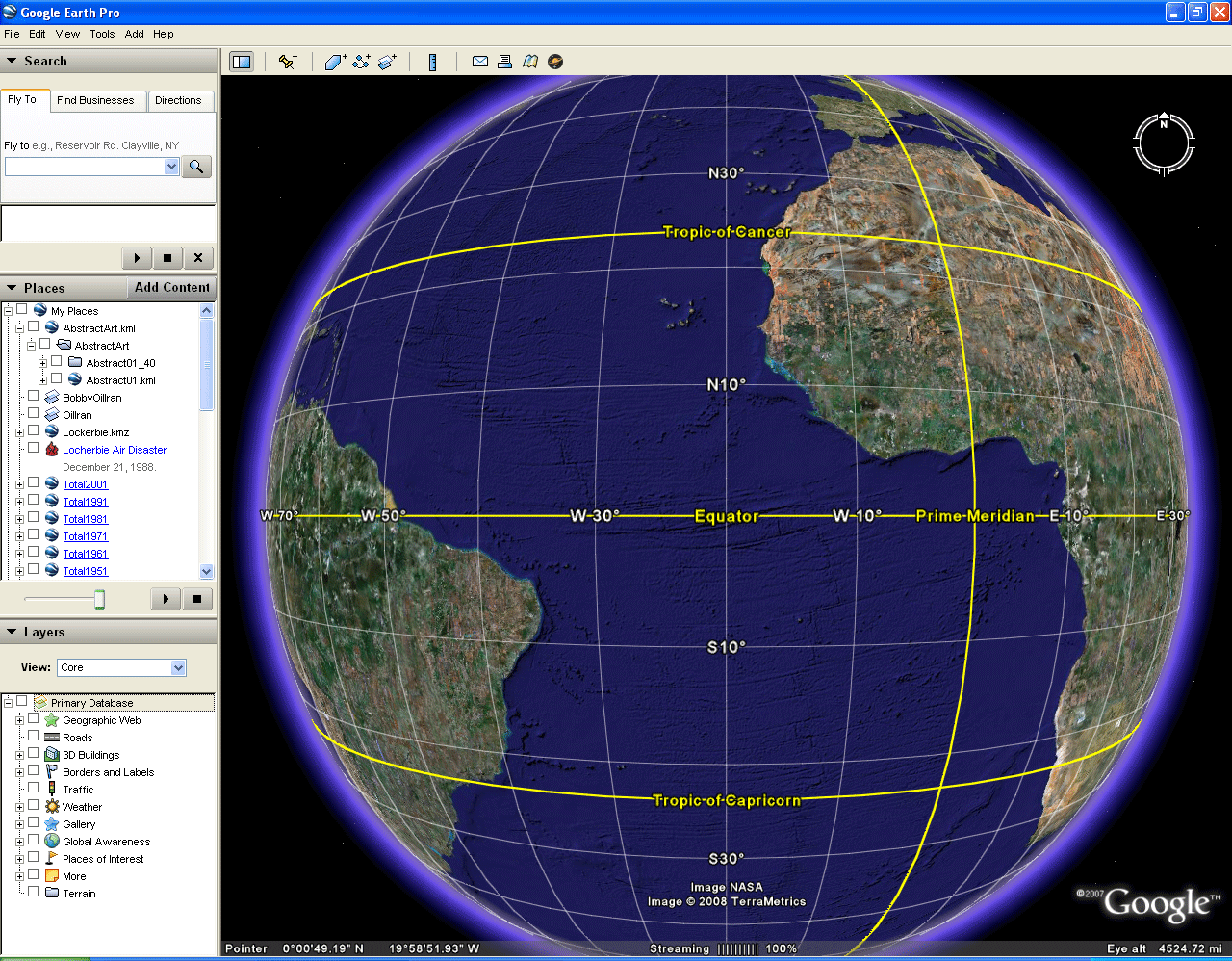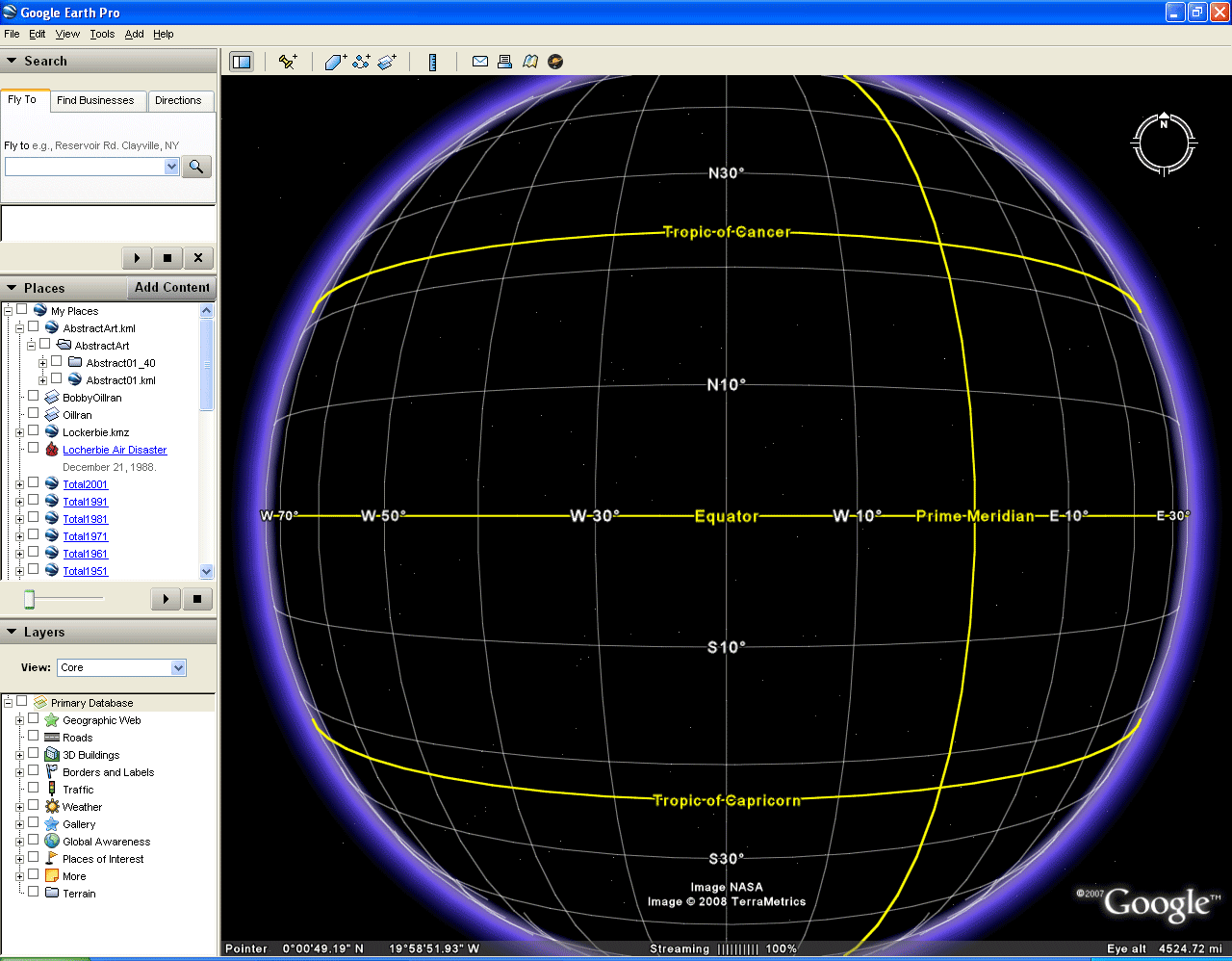 Figure 1. Unmasking Google Earth. |
| INSERTING
ABSTRACT
ART ON THE GOOGLE GLOBE In Figure 2, a simple repeating self-similar pattern is drawn using the lines of the graticule. Zoom in on the Google Earth graticule; finer partitions of latitude and longitude emerge making it easy to continue making smaller pattern. The sequence in Figure 2 illustrates how to execute such a process. |
 Figure 2. Inserting abstract art on the Google Globe. |
| TRANSPARENCY OF
THE GLOBE What is unclear from Figures 1 and 2 is whether one can look "through" the globe or whether the globe still has a skin on it that will block the simultaneous view of "front" and "back." When the Google Globe, with pattern from Figure 2, is set to spinning mode (drag it), it is clear that the globe, itself, is transparent. Watch as the generated pattern spins around a great circle in Figure 3 in an animated sequence of screen captures from Google Earth. Download the associated .kml flie linked here and try it yourself in Google Earth. In Figure 3, when the pattern alone is viewed going around the edge of the globe (from "front" to "back"), the black part of the pattern within the convex hull of the pattern (and a mat slightly beyond that hull) has become opaque and blocks out pattern behind it. This observation accounts for gaps in viewing the pattern as it spins around the great circle of Figure 3. To see this self-eclipsing effect of the pattern, try the downloaded kml file (best option) or view the animation as a movie. |
 Figure 3. Transparency of the Google Globe. Note the self-eclipsing effect causing the art to disappear as the Globe spins. |
| ECLIPSING
THE GOOGLE
GLOBE In the animation in Figure 4a, the black backing mat is quite clear as is the self-eclipsing effect. When the Earth's skin is returned to the globe (Figure 4a), along with the pattern, the black backing mat now become blue (when over the ocean). The mere return of the skin itself blocks the view through the globe; the white pattern that appears on the back side of the globe is completely blocked when the Earth skin, and even a very slightly opaque version of it, is returned to the Google Globe (Figure 4a). When height is assigned to a polygon, the self-eclipsing effect is removed. In Figure 4b, height is assigned to the yellow figure and its sides are extended to the ground. For contrast, the adjacent white figure is left on the ground. |
 Figure 4a. Transparency of the Pattern: Eclipsing the Google Globe. |
 Figure
4b.
Partial eclipse: assigning height removes much
of the
eclipsing effect. Download
associated .kml file.
|
| When a more solid
pattern, such as an Escher tile, is inserted on the
Google Globe (Figure 4c) it is clear that the method
by which the
tiling takes place conforms to the underlying
latitude/longitude
coordinate system (the method used to apply gores to
a globe). The
alignment of
Escher tiles is precise and easy to accomplish. A true tiling of a surface is one in which all tiles are the same size, independent of vantage point. Tiles on the horizon may appear to be small, but as one draws nearer, they reach full size. Geographer John Nystuen notes that he would also like to see increasing detail as one draws nearer. Because the Google Globe forces the use of the underlying coordinate system for tile alignment, no tiling of it that has north-south extent of more than one tile-width can ever be a true tiling; east-west tilings are true (Figure 4c). This fact may have serious implications for environmental and other studies that tile broad regions of the Earth with detailed maps or images. Self-eclipsing of tiles, be the pattern a true tiling or not, may be overcome by adding elevation to the tiles (Figure 4d). Care needs to be taken to size and color edge polygons of adjacent tiles so that the fit is good. Use the Four Color Theorem to reduce color clutter. |
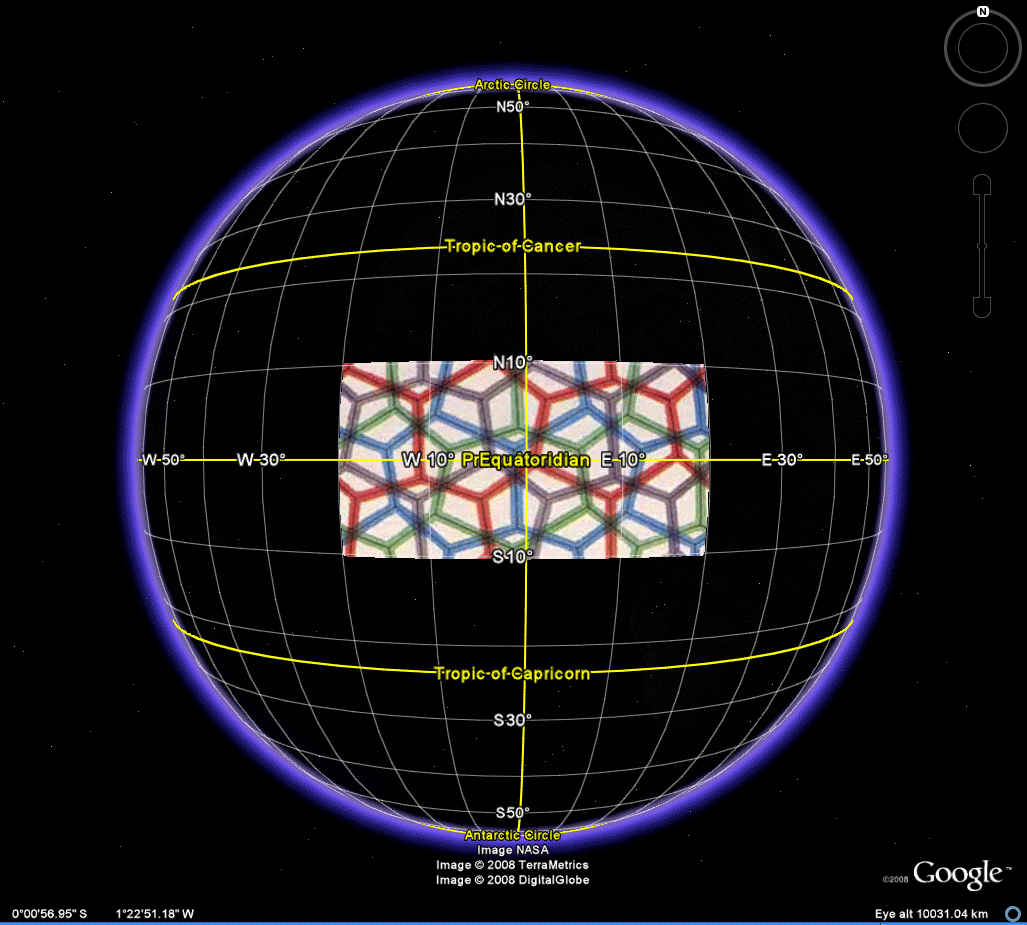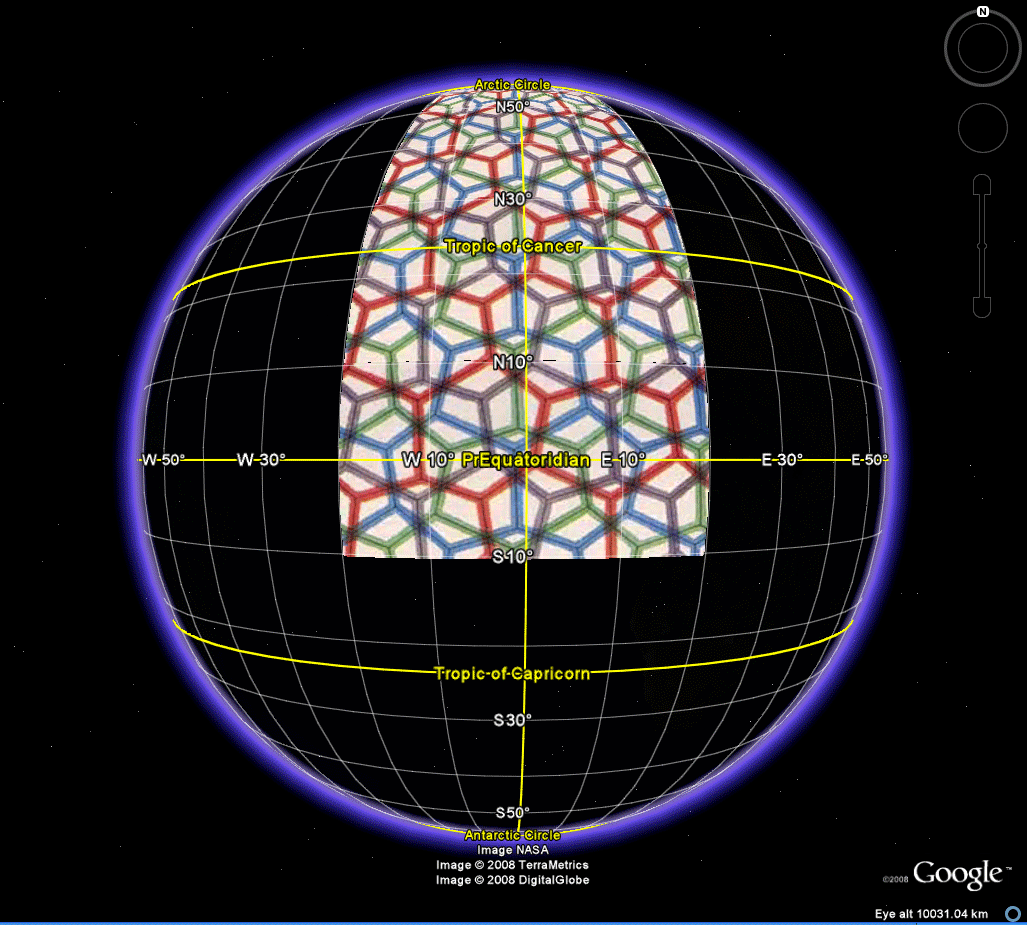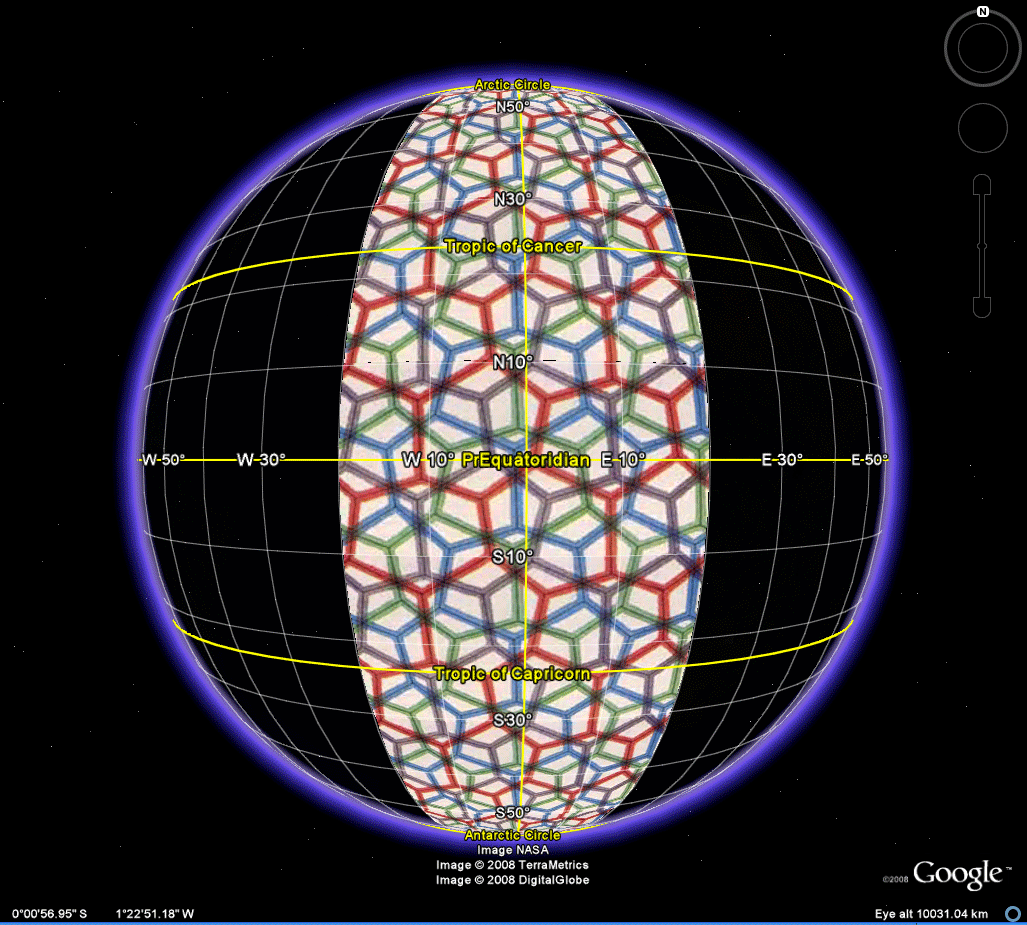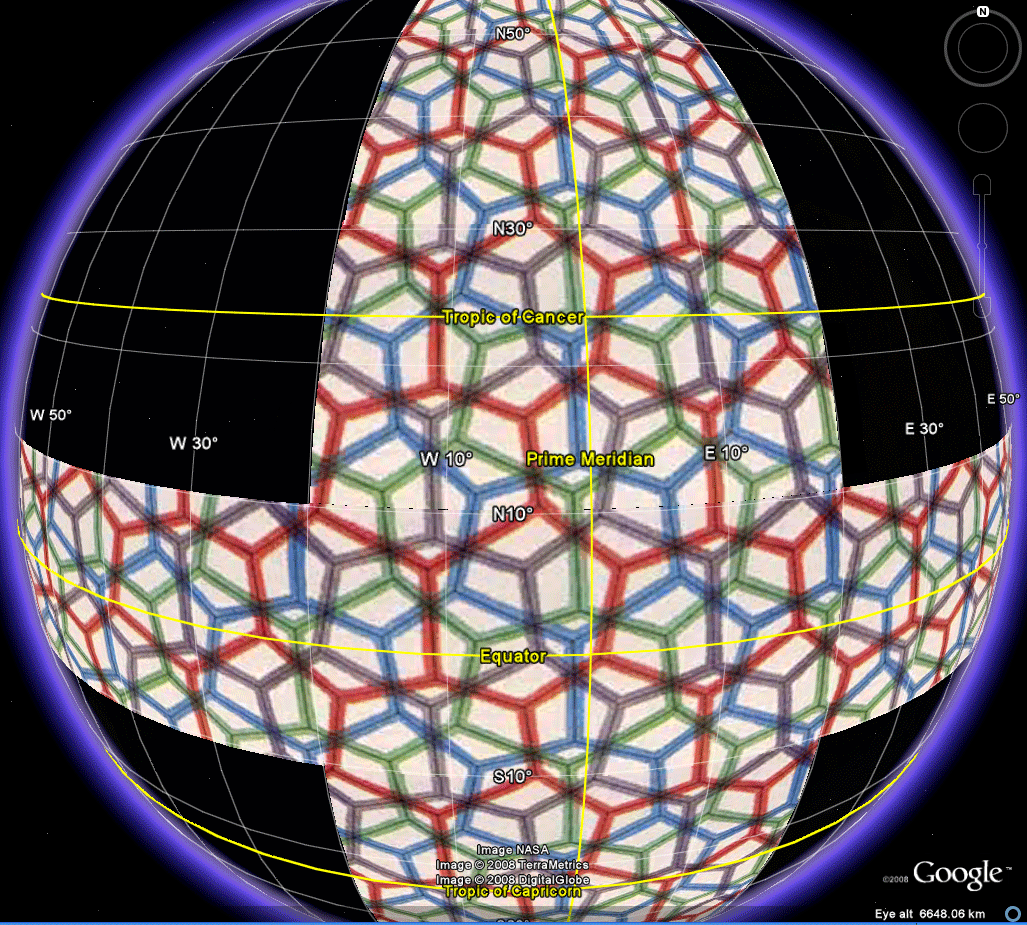 Figure
4c.
Tiling the Google Globe with Escher-like
tiles. The
globe gores
do not make a true tiling from north to south.
The east-west
pattern is true.
|
 Figure
4d.
Adding a third dimension to Escher tiles removes
self-eclipsing
effects. Size and color edge pieces
appropriately so that
adjacent
tiles will fit properly. Use of the Four Color
Theorem reduces
color clutter.
|
| DAVID
BARR'S FOUR CORNERS SCULPTURE One application that benefits greatly from the transparent globe effect is the visualization of David Barr's Four Corners Sculpture. In the late 20th century, Michigan sculptor David Barr set out to build an Earth-sized sculpture composed of small granite tetrahedra representing the vertices of an abstract tetrahedron inscribed in the earth-sphere. This project was more than a mere exercise. Barr actually travelled to four remote locations and planted the corners. He began in Easter Island, continued to the Kalahari Desert in southern Africa, went to the Greenland icecap, and ended up on a small island just off Irian Jaya/New Guinea in Indonesia. His adventure, which took many years, is chronicled in a variety of places including in film shot by a crew from the Archives of American Art (Smithsonian Institution) that traveled with him. The mathematical geography is archived in the work cited below by Arlinghaus and Nystuen. Barr has recently published (2007) a memoir in which more of the story is told. John Nystuen made the original calculations for Barr's corners in the 1970s. To do so, he used annular cardboard collars on a globe. Today, it is an easy matter to use placemarks in Google Earth to locate the positions where Barr's Corners are on the Earth. The "placemark" feature in Google Earth was used to pinpoint location by typing in exact latitude and longitude. Because placemarks do not show up on the back side of the Google Globe, polygons small enough not to experience any substantial self-eclipsing effect were also created at each Corner location. Figures 5a through 5d show a sequence of screen captures from the kml file associated with each of Barr's Four Corners. Download the associated .kml file. One evident advantage to using Google Earth, and to downloading the linked file, is that the reader can zoom in and get some modest appreciation for the surrounding terrain as well as for the degree of difficulty the artist must have encountered in placing the vertices in disparate locations! |
 Figure 5a. Barr and team began in Easter Island. They had surveying equipment of Thor Heyerdahl. There, the first movement of the Four Corners Ballet was danced by, among others, Barr's wife Beth Dwaihy Barr. |
 Figure 5b. The second vertex was planted on a farm, called "Karee Boom" in southern Africa. There the second ballet movement was danced as the vertex was planted in the Kalahari Desert. The ceremony was attended peacefully by both white and black Africans. |
 Figure 5c. The third vertex was planted on the Greenland icecap. As Barr left the site, a vicious storm formed around his tiny plane forcing him to jettison most of his equipment in order to survive. |
 Figure 5d. Despite the adversity encountered elsewhere, the Indonesian vertex proved, in the end, the most difficult. Repressive political problems forced interminable delays and eventually only an agreement to move the calculated location from the main island to a tiny island got Barr the permission he needed to finish his sculpture. |
| Visualizing this
scupture is difficult; all four vertices are
not simultaneously visible
on an opaque sphere. Figure 6, the
transparent Google Globe, shows all four
vertices simultaneously.
Rotate the Google Globe to produce different
patterns of the vertices in relation to the
hemispherical
horizon. |
 Figure 6. Download the associated .kml file. |
| Draw a path
to join vertices (Figure 7), as a graph with
edges lying
along arcs of great circles on the transparent
globe. The
tetrahedral nature of the Earth-sized
sculpture becomes clear.
Combining the various effects from above, in
the download (best option)
or in a linked
movie file,
produces a
visual summary and demonstrates the necessity
of transparency in
visualizing the sculptural whole--its physical
elements as well as its
story--as the sum of its parts. |
 Figure 7. The associated .kml file is contained within the download for Figure 6. |
REFERENCES:
- Arlinghaus,
Sandra
Lach. The
Animated Pascal, Solstice:
An
Electronic Journal of Geography
and Mathematics, Volume XVIII, Number 2, December
2007.
- Arlinghaus,
Sandra
and Batty, Michael. Zipf's
Hyperboloid? Solstice:
An
Electronic Journal of
Geography and Mathematics, Volume XVII,
Number 1,
June
2006.
- Arlinghaus, Sandra and Batty, Michael. Visualizing Rank and Size of Cities and Towns. Solstice: An Electronic Journal of Geography and Mathematics, Volume XVII, Number 2, December 2006.
- Part I: England, Scotland, and Wales, 1901-2001
- Part
II: Greater London, 1901-2001
- Arlinghaus,
Sandra
L. and Nystuen, John D. Sandra
L. Mathematical
Geography
and
Global
Art: the Mathematics of David Barr's `Four Corners
Project'.
Institute of
Mathematical Geography. 1986. Monograph
One
- Barr,
David. Crossing
Lines.
2007.
- Escher,
Maurits. Circle Limit Series.
- Pattern:
Lesson
7 Math Part--Escher. http://www.dartmouth.edu/~matc/math5.pattern/lesson7math.html
- Others
inline or
archived in Deep
Blue.
Dr. Arlinghaus is Adjunct Professor at The University of Michigan, Director of IMaGe, and Executive Committee Member, Community Systems Foundation.
Solstice: An Electronic
Journal
of Geography and
Mathematics,
Institute of Mathematical Geography, Ann Arbor, Michigan.
Volume XIX, Number 2.
http://www.InstituteOfMathematicalGeography.org/
Persistent URL: http://deepblue.lib.umich.edu/handle/2027.42/58219
Volume XIX, Number 2.
http://www.InstituteOfMathematicalGeography.org/
Persistent URL: http://deepblue.lib.umich.edu/handle/2027.42/58219